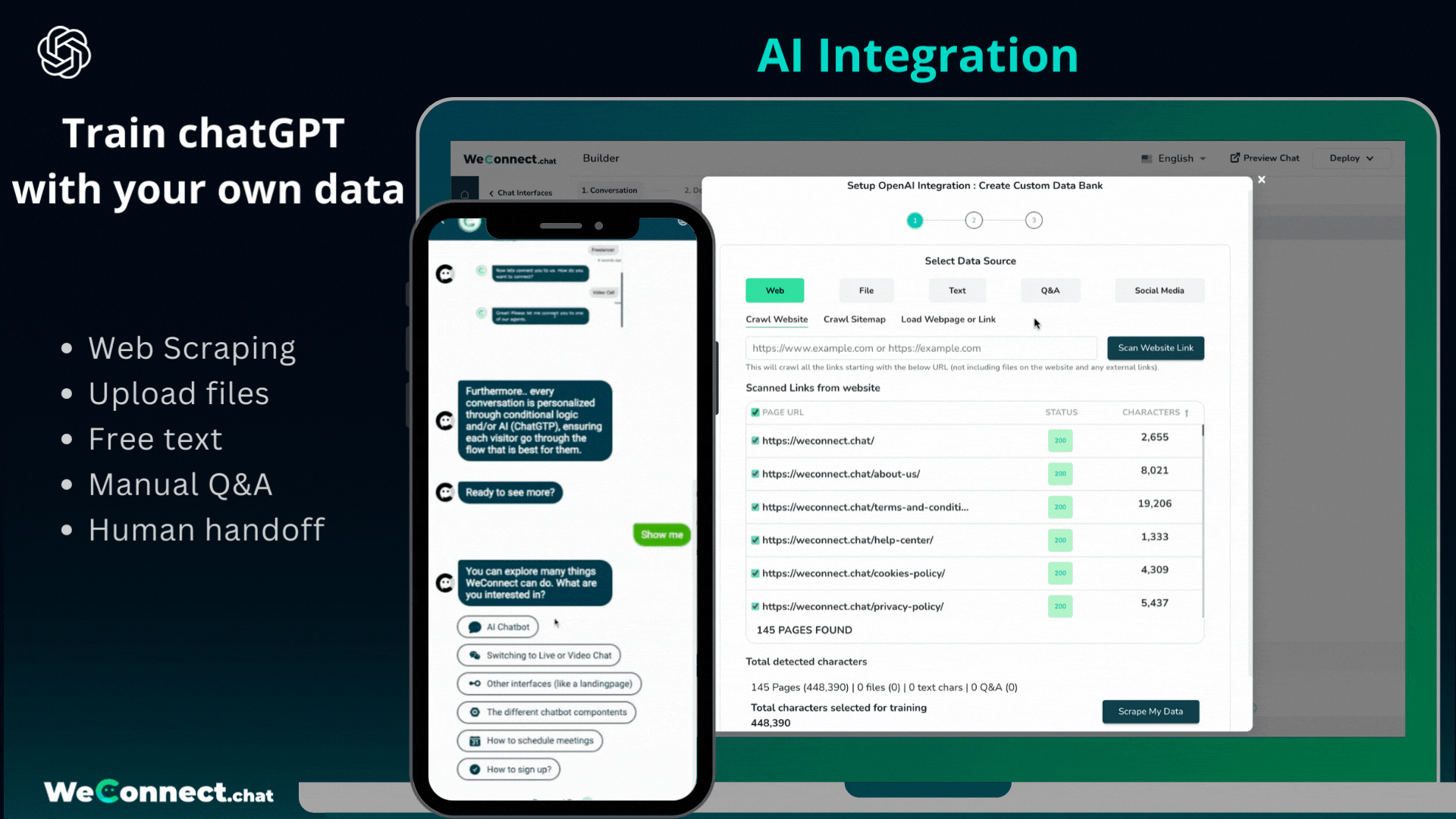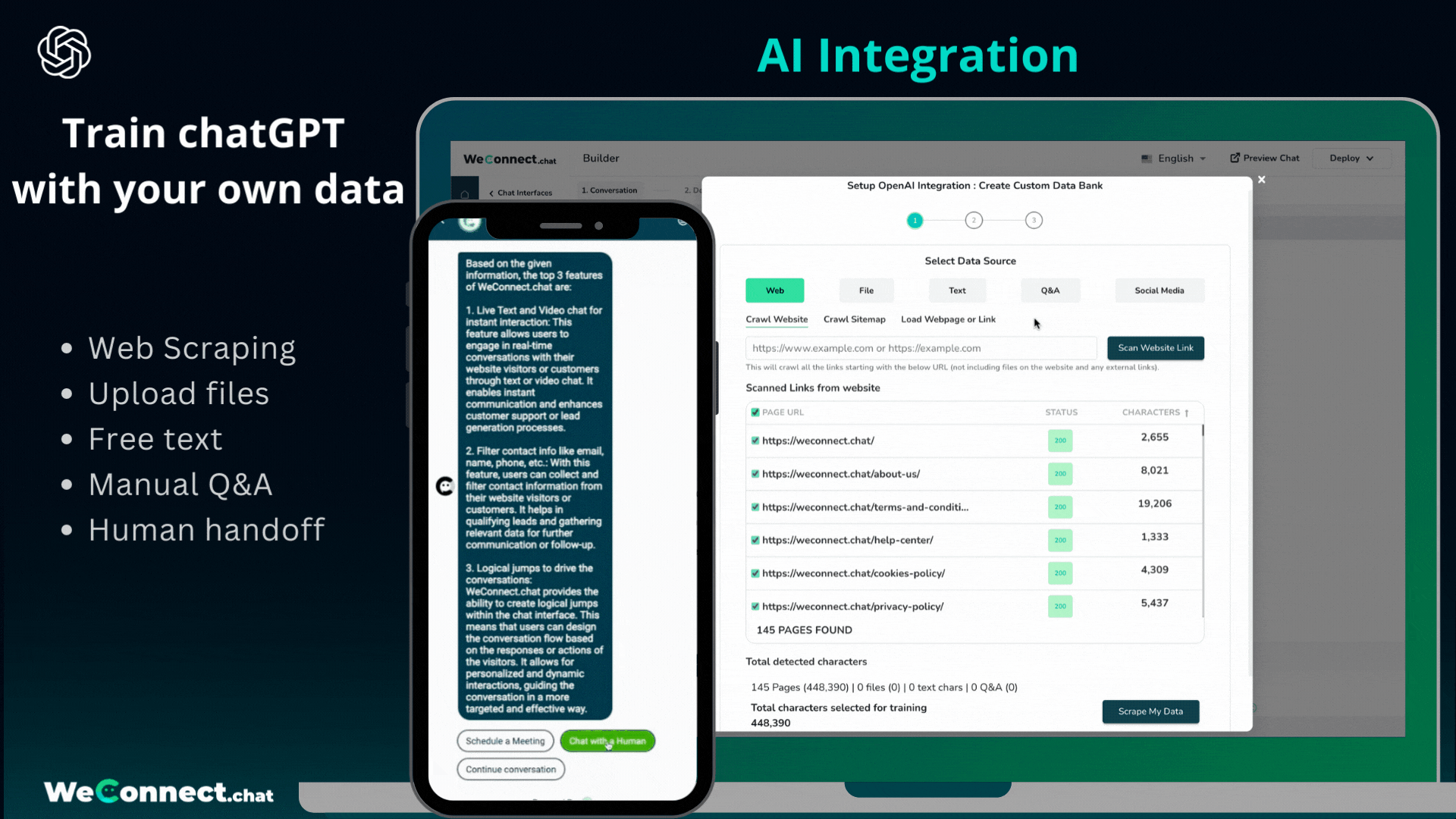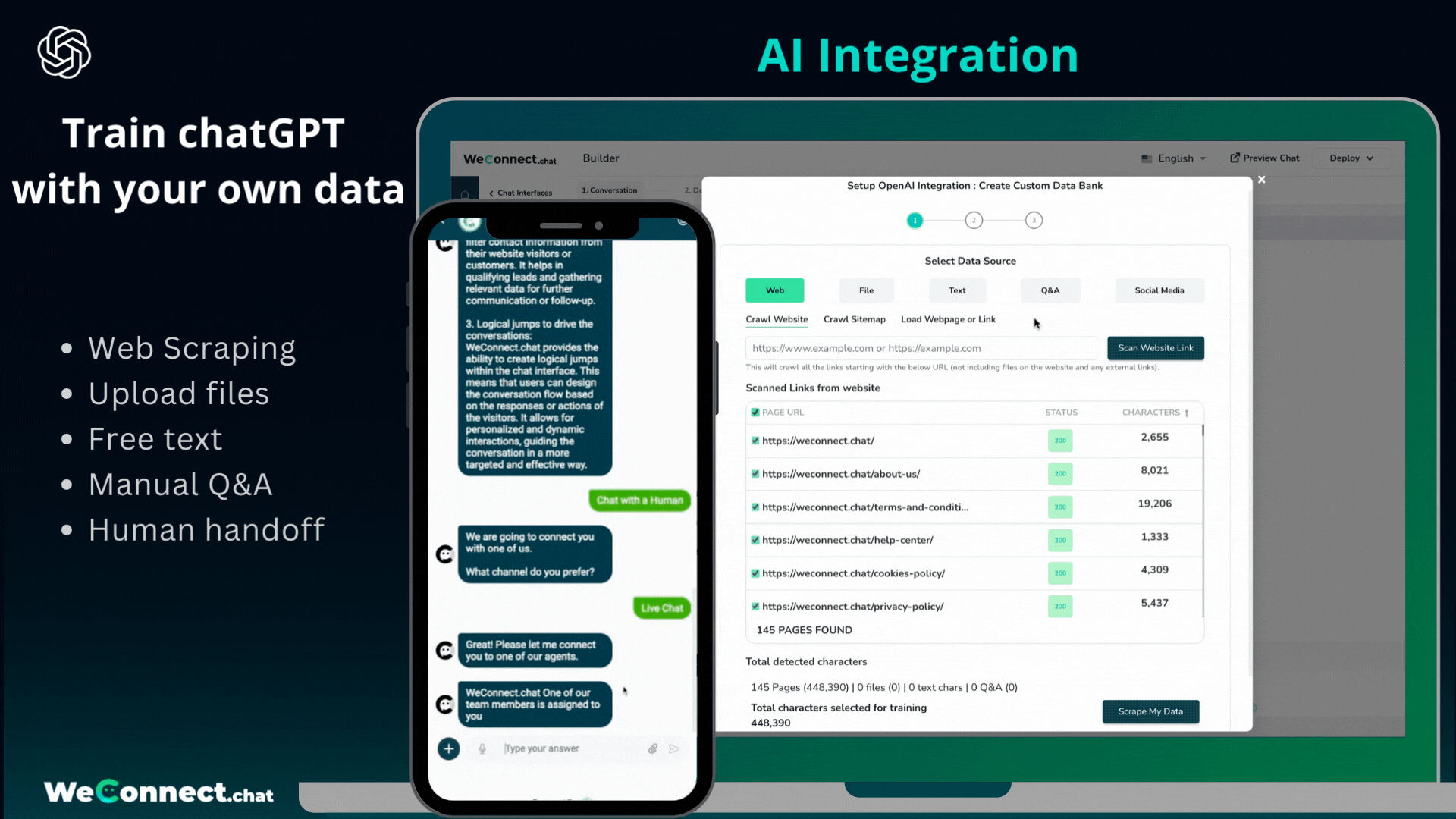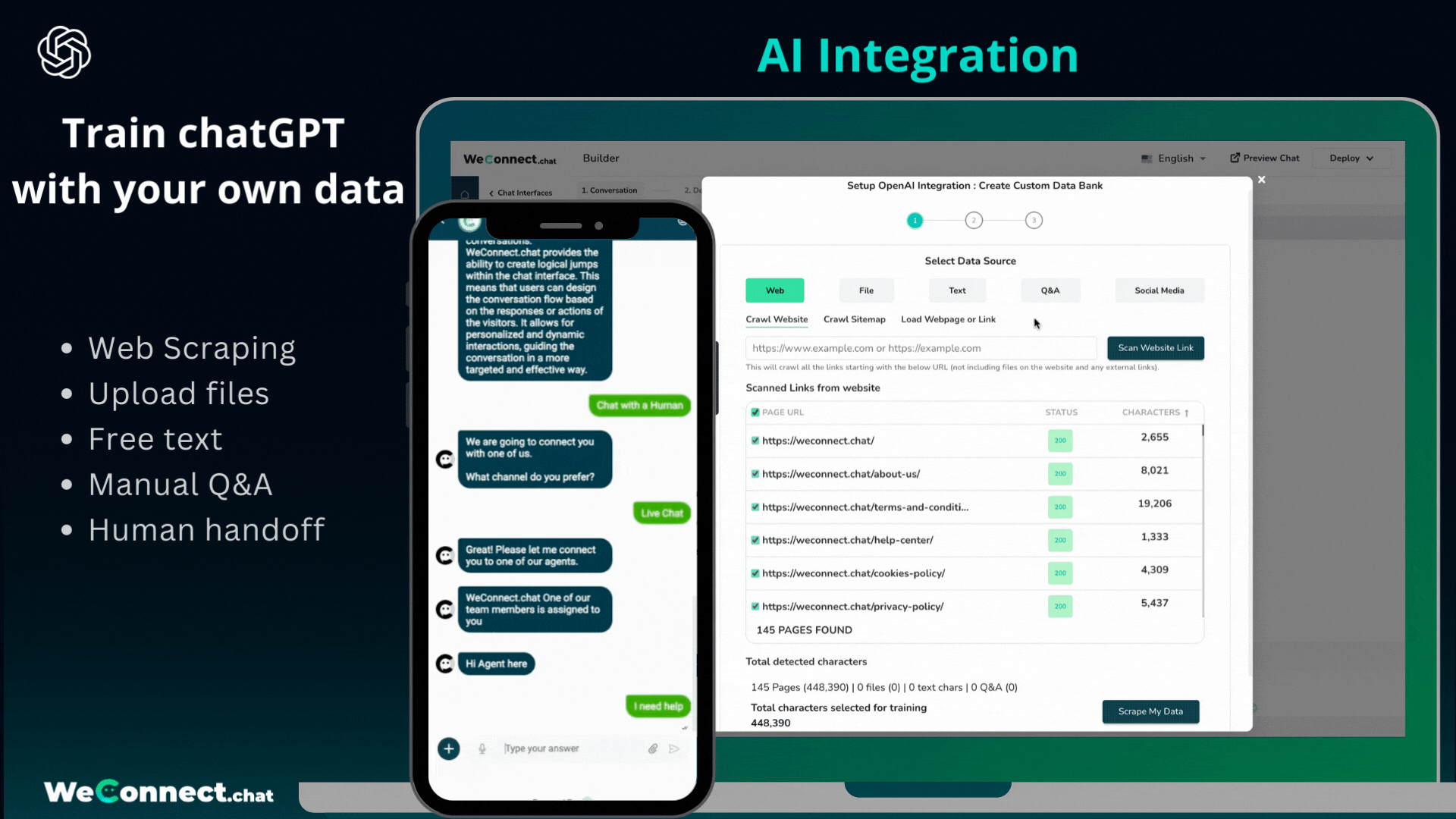
The integration of x ^ -1 is a fundamental concept in calculus, often encountered by students and professionals alike. Understanding this mathematical operation is crucial for solving a wide array of problems in fields such as physics, engineering, and economics. By mastering the integration of x ^ -1, one can gain insights into the behavior of logarithmic functions and their applications. The process of integrating x ^ -1 is linked to the natural logarithm, a key mathematical constant that appears in various scientific and engineering contexts. This connection provides a powerful tool for analyzing and interpreting complex systems, making the integration of x ^ -1 an essential skill for anyone interested in advanced mathematics.
In this comprehensive guide, we will delve into the intricacies of the integration of x ^ -1, exploring its theoretical foundations, practical applications, and common challenges. We will also provide step-by-step instructions for performing this integration, along with tips and tricks to help you avoid common pitfalls. Whether you are a student seeking to improve your calculus skills or a professional looking to apply these concepts in your work, this article will provide you with the knowledge and confidence you need to succeed.
By the end of this article, you will have a thorough understanding of the integration of x ^ -1 and its significance in various fields. We will cover everything from basic definitions and historical context to advanced techniques and real-world applications. So, let's dive into the world of calculus and unlock the full potential of the integration of x ^ -1.
Read also:Mastering The Art Of Social Sharing With Sharesnapchatcom For Unmatched Engagement
Table of Contents
- What is Integration?
- Historical Context of Integration
- Understanding x ^ -1
- The Fundamental Theorem of Calculus
- Basics of Integration
- Techniques for Integrating x ^ -1
- Connection to Logarithmic Functions
- Real-World Applications of x ^ -1 Integration
- Common Pitfalls and How to Avoid Them
- Step-by-Step Guide to Integrating x ^ -1
- Practice Problems and Solutions
- FAQs about Integration of x ^ -1
- Conclusion
What is Integration?
Integration is a fundamental concept in calculus, often described as the reverse process of differentiation. It involves finding the integral of a function, which represents the accumulation of quantities, such as area under a curve, total distance traveled, or the total accumulated change over a period. By integrating a function, we can determine the total outcome of a system described by that function over a specified interval.
Why is integration important?
Integration plays a crucial role in various fields, including physics, engineering, and economics, where it is used to solve problems involving rates of change, areas, and volumes. In physics, for example, integration helps calculate the work done by a force or the electric charge accumulated over time. In engineering, it is used to analyze stress and strain in materials, while in economics, it helps model consumer behavior and market dynamics.
Types of integrals
- Definite Integral: Represents the area under a curve over a specified interval, providing a finite value.
- Indefinite Integral: Represents a family of functions whose derivatives are equal to the original function, often expressed with a constant of integration, C.
Historical Context of Integration
The concept of integration has a rich history, dating back to ancient mathematicians like Archimedes, who used geometric methods to calculate areas and volumes. However, the formal development of integration as we know it today began in the 17th century, with the contributions of mathematicians like Isaac Newton and Gottfried Wilhelm Leibniz.
Who were the pioneers?
Newton and Leibniz are credited with independently developing the fundamental ideas of calculus, including differentiation and integration. Their work laid the foundation for modern analysis and led to significant advancements in mathematics and science.
Contributions of Newton and Leibniz
- Newton: Developed the method of fluxions, which involved the concept of instantaneous rates of change.
- Leibniz: Introduced the integral symbol (∫) and developed the notation for differentiation and integration still used today.
Understanding x ^ -1
The expression x ^ -1 represents the reciprocal of x, or 1/x. This simple yet powerful mathematical concept is essential for understanding the behavior of certain functions, especially those involving logarithms. When integrated, x ^ -1 yields the natural logarithm function, ln(x), which is a cornerstone of calculus and has numerous applications in science and engineering.
Why is x ^ -1 significant?
The integration of x ^ -1 is significant because it provides a direct connection to logarithmic functions, which are used to model exponential growth and decay, as well as to solve equations involving exponential relationships. This makes x ^ -1 a crucial component in understanding complex systems and phenomena.
Read also:Origins And Influence Of The Democraticrepublican Party In American Politics
Properties of x ^ -1
- Reciprocal: x ^ -1 is the reciprocal of x, meaning it is the inverse of multiplication.
- Domain: x ^ -1 is defined for all real numbers except zero, as division by zero is undefined.
The Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a pivotal result that links the concepts of differentiation and integration. It consists of two parts: the first part establishes the relationship between the derivative of an integral and the original function, while the second part provides a method for evaluating definite integrals using antiderivatives.
What is the significance of the Fundamental Theorem?
The Fundamental Theorem of Calculus is significant because it provides a systematic way to calculate integrals and solve calculus problems. It allows us to evaluate the accumulated change over an interval by finding the antiderivative of a function, simplifying the process of integration.
Components of the Fundamental Theorem
- Part 1: If F(x) is the antiderivative of f(x), then the derivative of the integral of f(x) with respect to x is f(x).
- Part 2: The definite integral of f(x) from a to b is equal to the difference in the values of its antiderivative, F(x), at b and a.
Basics of Integration
Mastering the basics of integration is crucial for solving complex calculus problems. These fundamentals include understanding the notation, rules, and techniques for finding integrals of various functions. By grasping these concepts, you will be better equipped to tackle more advanced topics, such as the integration of x ^ -1.
What are the key integration rules?
Several key rules form the foundation of integration, including the power rule, constant multiple rule, and sum rule. These rules help simplify the process of finding antiderivatives and are essential for solving a wide range of calculus problems.
Common Integration Rules
- Power Rule: ∫x^n dx = (x^(n+1))/(n+1) + C, for n ≠ -1.
- Constant Multiple Rule: ∫cf(x) dx = c∫f(x) dx, where c is a constant.
- Sum Rule: ∫(f(x) + g(x)) dx = ∫f(x) dx + ∫g(x) dx.
Techniques for Integrating x ^ -1
Integrating x ^ -1 requires a unique approach, as it does not fit the standard power rule. Instead, the integration of x ^ -1 results in the natural logarithm function, ln(x). Understanding this technique is essential for solving problems involving logarithmic relationships and exponential growth or decay.
How do you integrate x ^ -1?
To integrate x ^ -1, recognize that it is the derivative of the natural logarithm, ln(x). Therefore, the integral of x ^ -1 with respect to x is ln|x| + C, where C is the constant of integration.
Steps for Integrating x ^ -1
- Identify the function: Recognize that x ^ -1 is the reciprocal of x.
- Apply the integration rule: Use the fact that the integral of x ^ -1 is ln|x| + C.
- Simplify the expression: Ensure the result is expressed in terms of ln(x) to highlight the connection to logarithmic functions.
Connection to Logarithmic Functions
The integration of x ^ -1 is directly tied to logarithmic functions, specifically the natural logarithm, ln(x). This relationship is vital for understanding the properties and behavior of logarithmic functions, which are widely used in various scientific and engineering applications.
Why are logarithmic functions important?
Logarithmic functions are important because they model exponential growth and decay, which are common phenomena in nature and industry. They also provide a convenient way to solve equations involving exponential relationships, making them indispensable tools in many fields.
Applications of Logarithmic Functions
- Exponential Growth: Logarithmic functions describe phenomena like population growth and radioactive decay.
- Acoustics: Logarithms are used to measure sound intensity and decibel levels.
- Economics: Logarithmic scales help model economic growth and inflation rates.
Real-World Applications of x ^ -1 Integration
The integration of x ^ -1 has numerous real-world applications, particularly in fields that involve exponential relationships. By understanding this concept, professionals can better analyze and predict the behavior of complex systems, leading to more accurate models and solutions.
How is x ^ -1 integration used in real life?
In real life, the integration of x ^ -1 is used to model and analyze various phenomena, such as population growth, radioactive decay, and financial investments. By applying this concept, professionals can gain insights into the behavior of these systems and make more informed decisions.
Examples of Real-World Applications
- Physics: Calculating the time required for a radioactive substance to decay to a certain level.
- Finance: Modeling the growth of investments over time using compound interest formulas.
- Biology: Analyzing population dynamics and predicting future growth trends.
Common Pitfalls and How to Avoid Them
While integrating x ^ -1 is a straightforward process, there are several common pitfalls that students and professionals may encounter. By being aware of these challenges and knowing how to avoid them, you can ensure accurate and reliable results.
What are the common mistakes in x ^ -1 integration?
Some common mistakes in x ^ -1 integration include misapplying the power rule, forgetting the constant of integration, and misunderstanding the connection to logarithmic functions. By understanding these errors, you can take steps to prevent them in your work.
Tips for Avoiding Common Pitfalls
- Remember that x ^ -1 is the reciprocal of x, not a power function.
- Always include the constant of integration, C, in indefinite integrals.
- Recognize the connection between x ^ -1 and logarithmic functions, ensuring your results are expressed in terms of ln(x).
Step-by-Step Guide to Integrating x ^ -1
This step-by-step guide will walk you through the process of integrating x ^ -1, providing clear instructions and helpful tips to ensure success. By following these steps, you can confidently tackle problems involving this integration and achieve accurate results.
How to integrate x ^ -1 step by step?
Integrating x ^ -1 involves a straightforward process that relies on recognizing its connection to logarithmic functions. By following these steps, you can accurately perform this integration and apply it to various problems.
Steps for Integrating x ^ -1
- Identify the function: Recognize that you are working with x ^ -1, the reciprocal of x.
- Apply the integration rule: Use the fact that the integral of x ^ -1 is ln|x| + C.
- Simplify the expression: Ensure the result is expressed in terms of ln(x) and includes the constant of integration, C.
- Verify your work: Double-check your calculations and ensure the result is consistent with the properties of logarithmic functions.
Practice Problems and Solutions
To solidify your understanding of the integration of x ^ -1, it's essential to practice solving problems. This section provides several practice problems, along with detailed solutions, to help you hone your skills and gain confidence in your abilities.
What are some practice problems for x ^ -1 integration?
The following practice problems will challenge your understanding of x ^ -1 integration and help you apply the concepts covered in this article. By working through these problems, you can reinforce your knowledge and improve your problem-solving skills.
Practice Problems
- Integrate the function f(x) = 1/x over the interval [1, 5].
- Find the indefinite integral of g(x) = 1/x with respect to x.
- Evaluate the definite integral of h(x) = 1/x from 2 to 6.
Solutions
- Using the definite integral formula, ∫[1,5] 1/x dx = ln|5| - ln|1| = ln(5).
- The indefinite integral of g(x) = 1/x is ln|x| + C.
- The definite integral of h(x) = 1/x from 2 to 6 is ln|6| - ln|2| = ln(3).
FAQs about Integration of x ^ -1
Here are some frequently asked questions about the integration of x ^ -1, along with concise answers to help clarify common concerns and misconceptions.
What is the integral of x ^ -1?
The integral of x ^ -1 is ln|x| + C, where C is the constant of integration.
Why can't I use the power rule for x ^ -1?
The power rule does not apply to x ^ -1 because the exponent is -1, which leads to division by zero when using the formula for antiderivatives. Instead, recognize that x ^ -1 is the derivative of ln(x).
How does the integration of x ^ -1 relate to logarithms?
The integration of x ^ -1 results in the natural logarithm function, ln(x), highlighting the connection between reciprocal functions and logarithms.
What are some real-world applications of x ^ -1 integration?
Real-world applications include modeling population growth, radioactive decay, and financial investments, where exponential relationships are involved.
Can I integrate x ^ -1 over any interval?
You can integrate x ^ -1 over any interval that does not include zero, as division by zero is undefined.
What are the common mistakes to avoid when integrating x ^ -1?
Common mistakes include misapplying the power rule, forgetting the constant of integration, and misunderstanding the connection to logarithmic functions.
Conclusion
In conclusion, mastering the integration of x ^ -1 is an essential skill for anyone interested in advanced mathematics, physics, engineering, or economics. By understanding the theoretical foundations, practical applications, and common challenges associated with this integration, you can confidently tackle complex problems and gain valuable insights into the behavior of logarithmic functions.
Through this comprehensive guide, we have explored the intricacies of the integration of x ^ -1, providing step-by-step instructions, practice problems, and solutions to help you hone your skills. By applying this knowledge in real-world scenarios, you can enhance your problem-solving abilities and make more informed decisions in your chosen field.
We hope this guide has provided you with a deeper understanding of the integration of x ^ -1 and its significance in various contexts. As you continue to explore the world of calculus, remember to practice regularly, seek out new challenges, and never stop learning. The possibilities are endless, and with dedication and perseverance, you can achieve great success in your mathematical journey.
For further reading on the topic of logarithmic functions and their applications, consider exploring external resources such as Khan Academy's Calculus Course, which offers a wealth of information and practice exercises to enhance your understanding of calculus concepts.